I've been doing homework for hours today and seem to be held up on two questions. If any one can reply with answers to these questions by the end of tonight (assignment is due tomorrow morning), and more importantly how you got the answers, I'll donate 2,000 slappin' points.
Question 1:
2x^4+3x^3-x+1 divided by x^2+x-1
Question 2: List the possible rational zeros of the function. Then find all the zeros of the function.
f(x)=3x^3+10x^2-7x-20
Board index ‹ Off Topic Board ‹ Off Topic Discussion ‹ Math Help?? 2,000 slappin' points
Math Help?? 2,000 slappin' points
13 posts
• Page 1 of 1
The answer is:
2x^2+x+1 with a remainder of -x+2
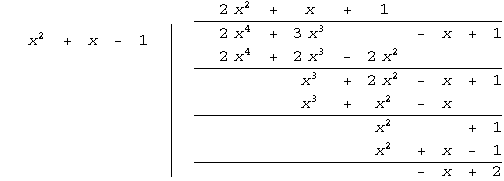
http://calc101.com/index.html
This is a very good site with anything math related if you need future help. It shows you every step and should hopefully help you learn it. This problem was obviously long division, I wish I would have found it before I worked it out![test [lol]](https://www.coastercrazy.com/forums/images/smilies/icon_e_ugeek.gif)
2x^2+x+1 with a remainder of -x+2
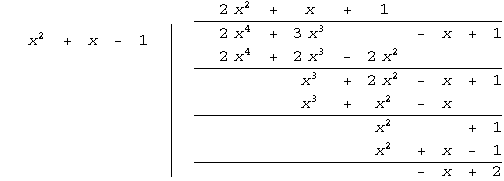
http://calc101.com/index.html
This is a very good site with anything math related if you need future help. It shows you every step and should hopefully help you learn it. This problem was obviously long division, I wish I would have found it before I worked it out
"and more importantly, how you got the answer"
Thanks Lewis, but what about question 2?
For the second one set f(x)=0 and solve for x.
Possible rational zeros of the function:
?????????1
?????????2
?????????4
?????????5
?????????10
?????????20
These are written as a fraction over 3, incase you're confused, the plus minus sign is for both numbers so put it out in front of the fraction to the left.
?????????1/3
????????? 2/3
?????????4/3
?????????5/3
?????????10/3
????????? 20/3
Tutorial Can Be Found Here: http://www.wtamu.edu/academic/anns/mps/ ... _zero1.htm
________________________________
I'm not sure about the second part, I'll try to find out more information I'm not really experienced with this stuff but I know that the first part is right. Not bad for a 15 year old eh.
?????????1
?????????2
?????????4
?????????5
?????????10
?????????20
These are written as a fraction over 3, incase you're confused, the plus minus sign is for both numbers so put it out in front of the fraction to the left.
?????????1/3
????????? 2/3
?????????4/3
?????????5/3
?????????10/3
????????? 20/3
Tutorial Can Be Found Here: http://www.wtamu.edu/academic/anns/mps/ ... _zero1.htm
________________________________
I'm not sure about the second part, I'll try to find out more information I'm not really experienced with this stuff but I know that the first part is right. Not bad for a 15 year old eh.
Ahh, thanks so far Lewis. Yeah, not bad for 15. Haha, I'm 16 but in college.
To find all the possible rational zeros of the function, you have to take the factors of the leading coefficient and divide them by the factors of the constant in the problem.
This means that since the factors of 3 are -1 +1 -3 and +3
The factors of 20 are -1, 1, -2, 2, -4, 4, -5, 5, -10, 10, -20, and 20
I posted the answer above hopefully you somewhat get how I got it. Part two is confusing me to death though.
This means that since the factors of 3 are -1 +1 -3 and +3
The factors of 20 are -1, 1, -2, 2, -4, 4, -5, 5, -10, 10, -20, and 20
I posted the answer above hopefully you somewhat get how I got it. Part two is confusing me to death though.
I'm checking out that link, and yeah part two is confusing..
^You've already done the difficult part, now it's just a matter of picking out the ones that actually work for the function, remember that the method you used only gives every possible rational root (only a handful these actually ARE roots of the given equation). Looks to me like -4/3 is the only rational root of this equation. To see the solution in action, just plug in -4/3 for x and it'll go to zero. This won't work for any of those other values.
Edit: If you'd like to "cheat" on this and use a fun bit of math history, you could apply Cardano's solution to it: http://en.wikipedia.org/wiki/Cubic_func ... 27s_method
Edit: If you'd like to "cheat" on this and use a fun bit of math history, you could apply Cardano's solution to it: http://en.wikipedia.org/wiki/Cubic_func ... 27s_method
Oh, so Kevin you're saying that now all you have to do is sort through the possible zeros and find the one that sets the function to zero?
Dang, i was doing something completely wrong lol.
Dang, i was doing something completely wrong lol.
^That's exactly it!
Factoring isn't going to happen
Actually, I'm retarded. This thing factors nicely into (3*x + 4)*(x^2 + 2*x - 5). From there all you need to do is figure out when x^2+2x-5 equals zero, because that will drive the entire expression to zero regardless of what the first part is (we already saw that one of the solutions is -4/3, which drives that part to zero). Anyhow, using the quadratic equation gives you an irrational pair of solutions, -1 +/- sqrt(6).
Edit: Well, I'm sick and heading to bed early. To summarize the second problem:
Given problem: 3*x^3 + 10*x^2 - 7*x - 20 = 0
Factor into: (3*x + 4)*(x^2 + 2*x - 5) = 0
Set each part equal to zero:
(3*x + 4) = 0
(x^2 + 2*x - 5) = 0
AND solve for x in each case. The first one is straightforward and gives you x = -4/3. The second one is probably best handled by smashing it with the quadratic formula, which you ought to know well when taking any algebra course This gives you x = -1 +/- sqrt(6), where "sqrt" means square root.
This gives you x = -1 +/- sqrt(6), where "sqrt" means square root.
x = -4/3, -1 + sqrt(6), -1 - sqrt(6)
Actually, I'm retarded. This thing factors nicely into (3*x + 4)*(x^2 + 2*x - 5). From there all you need to do is figure out when x^2+2x-5 equals zero, because that will drive the entire expression to zero regardless of what the first part is (we already saw that one of the solutions is -4/3, which drives that part to zero). Anyhow, using the quadratic equation gives you an irrational pair of solutions, -1 +/- sqrt(6).
Edit: Well, I'm sick and heading to bed early. To summarize the second problem:
Given problem: 3*x^3 + 10*x^2 - 7*x - 20 = 0
Factor into: (3*x + 4)*(x^2 + 2*x - 5) = 0
Set each part equal to zero:
(3*x + 4) = 0
(x^2 + 2*x - 5) = 0
AND solve for x in each case. The first one is straightforward and gives you x = -4/3. The second one is probably best handled by smashing it with the quadratic formula, which you ought to know well when taking any algebra course
x = -4/3, -1 + sqrt(6), -1 - sqrt(6)
Thanks for the help everyone
13 posts
• Page 1 of 1
Return to Off Topic Discussion
-
- Related topics
- Replies
- Views
- Last post
-
- need 500 slappin' points
by RideWarriorNation » July 14th, 2012, 8:09 pm - 12 Replies
- 2118 Views
- Last post by Coasterkat
July 16th, 2012, 1:35 pm
- need 500 slappin' points
-
- 14 Slappin points for the first person to...
by coasterboaster » September 4th, 2005, 2:37 pm - 8 Replies
- 1398 Views
- Last post by SFMM homie
September 5th, 2005, 4:02 pm
- 14 Slappin points for the first person to...
-
- Any love for Math rock?
by tmv8888 » February 18th, 2013, 12:36 pm - 0 Replies
- 593 Views
- Last post by tmv8888
February 18th, 2013, 12:36 pm
- Any love for Math rock?
-
- Slappin Happening
1, 2, 3, 4by Oscar » July 16th, 2003, 7:33 pm - 87 Replies
- 8592 Views
- Last post by Angry_Gumball
August 14th, 2003, 12:46 pm
- Slappin Happening
-
- Not getting points
by masterchief101 » May 9th, 2004, 9:31 am - 6 Replies
- 1393 Views
- Last post by hsxlc
May 10th, 2004, 7:50 pm
- Not getting points


